The Logic Class: Part 2

| JHDL Home Page | Configurable Computing Lab | User's Manual (TOC) |

The Logic Class: Part 2 - Technology Mapping and Placement in JHDL

Overview

Mapping: Relating logic to primitive FPGA blocks
Placement: Determining the location of primitive blocks on the FPGA Modifying the default Mapping and Placement is done with the goal of achieving higher speed, smaller area, and less logic (by using carry logic.)
Warning:: Mapping and placement were used extensively with the Xilinx XC4000 and Virtex families. Little, if any mapping and placement exist in the Virtex2 libraries. Thus, this may or may not be applicable to that technology.

Logic Class Part 2 Index

Traditional Mapping and Placement

JHDL Mapping and Placement

Logic placement and technology-mapping

Logic technology-mapping

Iterated Mapping

Logic Placement

Cell Transformations

Relational Port Placement

Summary

Introduction

What is Mapping and Placement?

"Mapping" is the term used to describe the grouping of logic together in a primitive FPGA block. For example, mapping logic in the Xilinx XC4000 architecture groups the logic into the LUTs and flip-flops of a CLB. In the Chess architecture, the logic would be grouped into the ALUs and memory blocks.

"Placement" involves grouping the primitive logic blocks on an FPGA. For example, placement in the Xilinx XC4000 architecture involves grouping the previously mapped LUTs and flip-flops into the appropriate CLBs and grouping the CLBs together on the FPGA. "Relative placement" refers to placing LUTs, flip-flops and CLBs relative to each other, and "absolute placement" refers to placing LUTs, flip-flops and CLBs at absolute locations of the FPGA.

Mapping and Placement will always be done during the back-end compilation step by automated map and PAR tools. However, if a user wishes to customize or optimize how circuitry is mapped or placed together, manual mapping and placement can be done during the design entry stage. For the rest of this manual, mapping and placement refer to the manual mapping and relative placement done during the design entry stage of circuit design.

Background and Motivation

Mapping and Placement of Circuitry in FPGAs

There are many desirable effects of mapping and placement within FPGAs. Mapping and placement can:

Decrease circuit area
Group related logic together in a CLB, ALU, or other primitive unit.
Decrease signal delay.

This is especially true in FPGAs where routing delay is greater than circuit delay.

Increase clock frequencies.
Preserve regularity
Utilize specialized data path features such as special carry-logic
Standardize interfaces
Decrease compile time

This is generally true because the back-end compilation tools for many technologies operate on Relationally Placed Macros (RPMs) instead of gates.

Although there are many advantages to manual mapping and placement, a hybrid solution between completely manual and completely automated placement usually produces the best results.

Traditional Mapping and Placement in FPGAs is difficult

Traditionally, designing FPGA-based circuits was done in VHDL or using a schematic entry tool like Viewlogic. While Placement in VHDL can be done through comments, it has many short-comings, namely:

Structural code and placement comments use different syntaxes
Parameterization is difficult. (this usually requires C code)
It is very verbose
It requires long design cycles

Manual placement can also be done in most schematic entry tools by attaching property strings to cells. However, this method of design and placement has many short-comings as well:

It is very verbose
It usually offers no programmatic functionality
It doesn't allow for parameterization
It requires long design cycles

JHDL Technology Mapping and Placement

JHDL was created to address many of difficulties with traditional FPGA mapping and placement. In particular, JHDL

Integrates placement code and structural design code in a programmatic high level language (JAVA).
Offers users fine-grained control through the TechMapper.
Offers convenience methods for the common cases to reduce verbosity (found in Logic).
Gives designers large amounts of flexibility.
Provides relational placement methods to reduce complexity.
Provides layout manipulation methods which reduce redesign time.
Allows error checking to be done as early as possible.

Technology-mapping and Logic placement

In JHDL it is suggested that all designs extend Logic to take advantage of its extensive technology independent methods. The Logic class implements two basic methods for specifying technology-specific mapping and placement: map() and place(). A simple example consists of the following code:

     Wire out = and( a, b );
     map(a, b, out);
     place( out, 2, 5 );

The first line creates an AND gate with a and b as inputs and out as the output. The second line maps a and b as inputs to a 4-LUT with out as the output. The last line places the the AND gate at the relative location (2,5). Note that in this example, the output wire was the object being placed. In JHDL, calling place() on a reference to the cell or on the output wire of the cell have the same effect--both result in the placement of the cell that drives the output wire.

We will give a more detailed explanation of the map() and place() calls later in the tutorial.

Technology Mapping through Logic

The map() call implies the mapping of a logic function to a target-specific "atomic cell". The "atomic cells" are LUTs and flip-flops for the XC4000 architecture, ALUs and flip-flops for the HP Chess architecture, etc. The map() call accepts a list of input wires and a single output wire; this list of wires implies the function that is to be mapped. For example, if we call:

     Wire out = or( and(a,b), and(a,ci), and(b,ci) );
     map( a, b, ci, out );

this implies mapping the function of the three AND gates and one OR gate into a single LUT in XC4000. The map() call currently accepts from 2 to 9 input parameters, and optionally accepts a String that gives target-specific constraints to the platform-specific TechMapper. See the documentation for your specific FPGA architecture for a list of possible constraints.

It is important to note that the Logic API defines absolutely nothing about the exact implementation of the map() call, beyond what has been specified here. The optional String arguments accepted by map() are specifically "defined to be undefined" in the Logic class! They receive no interpretation in the Logic class, and are passed straight to the TechMapper for platform-specific interpretation.

Technology mapping can be done explicitly by instantiating individual primitives. In the case of a full-adder in XC4000 we would write.

     or_o(and(a,b), and(a, ci), and(b, ci), sum);
     xor_o(a, b, ci, co);
     fmap f1 = new fmap(this, a, b, ci, sum);
     fmap f2 = new fmap(this, a, b, ci, co );

However, this limits technology independence since fmaps are specific to Xilinx Parts. The same thing can be achieved by targeting the XC4000TechMapper and implementing the fmaps through the Logic map() method. Using the Logic map() call still requires a knowledge of how logic is packed together into the basic FPGA elements. For example, the adder would be mapped to 2 LUTs in Xilinx XC4000. With this knowledge, the Logic methods look like this:

     or_o(and(a,b), and(a, ci), and(b, ci), sum);
     xor_o(a, b, ci, co);
     map(a, b, ci, sum);
     map(a, b, ci, co);

The above map methods will pass all the associated information to the TechMapper.

NOTE: While some error checking is done in the techmapper, users are advised to learn the restrictions of a technology before attempting to use the map method. For example, if you are trying to pack the logic into a 4-LUT in Xilinx XC4000, it doesn't make sense to use a map() call with nine input wires unless it is clear to the TechMapper how the nine inputs can be distributed among the various LUTs. For example, the following code would be valid in XC4000 since the 4-input and-gate and xor-gate can be mapped to the F-LUT and G-LUT, while the 3-input or-gate can be mapped to the H-LUT.

     Wire out = reg( or( a, and(b,c,d,e), xor( and(f,g), or(h,i) ));
     map( a,b,c,d,e,f,g,h,i, out );

Iterated Map

For the convenience of the user, map methods will accept multi-bit wires and iteratively map the individual bits of the input and output wires. Again, whether a particular group of wires is a valid mapped network depends on the techmapper being used. If we wanted to map a 5-bit psuedo-adder we could use the following code in Logic:

     Wire a = wire(5);
     Wire b = wire(5);
     Wire ci = wire(5);
     Wire co = wire(5);
     Wire sum = wire(5);
     or_o(and(a,b), and(a, ci), and(b, ci), sum);
     xor_o(a, b, ci, co);
     map(a, b, ci, sum);
     map(a, b, ci, co);

Each map method in this case would create 5 fmaps for the XC4000 architecture.

For most technologies, the wire widths in a map() call must be any combination of the atomic width or a single "generic" width. For example, in the Xilinx XC4000 architecture the following set of wire widths would be valid: { 1, 8, 8, 1, 8 }. On the other hand, the following set would be invalid, because there is more than one "generic" width: { 1, 8, 4, 1, 8 }. (Basically, in this case "generic" means anything other than 1-bit).

Logic Map methods return the new mapping cell or the driver of the output wire for future use in placement or layout manipulation.

Logic Placement

In general, JHDL placement in done by annotating placement hint strings onto Cells in a netlist. These hints communicate information to the back-end tools about where each cell is to be placed. For example, Xilinx uses a 2-d array of Logic blocks arranged in Rows and Columns. Each coordinate in the grid represents a CLB, with ".F" ".G", ".H", ".FFX", and ".FFY" representing the associated LUTs and flip-flops of the CLB.

Placement "hints", or properties, may be specified in many different ways. The most basic method, which allows users very fine-grained control without JHDL intervention, is to use the addProperty() method. To place the 1-bit full adder of the previous example in XC4000, we could use the following code:

    or_o(and(a,b), and(a, ci), and(b, ci), sum);
    xor_o(a, b, ci, co);
    map(a, b, ci, sum).addProperty("RLOC", "R0C0.F");
    map(a, b, ci, co).addProperty("RLOC", "R0C0.G");

This will append the property string "RLOC=R0C0.F" onto the fmap returned by the map method for the add logic, and append the property string "RLOC=R0C0.G" onto the fmap for the carry logic.

The place() method in Logic is designed to be a convenient way to append placement hints onto a cell. Placement hints can be added directly to cells without using an RLOC string by doing the following:

    place(Cell Sum, "R0C0.F");
    place(Cell Carry, "R0C0.G");

The place() calls simply attach an RLOC property with the given value to the specified Cells.

In order to preserve some notion of technology independence for placement, a generic grid of X and Y coordinates is used in Logic and interpreted by each TechMapper. At netlist time these X and Y coordinates, together with their hints, are converted into properties like the RLOCs for XC4000 and attached to the appropriate cells. The JHDL placement grid has Logic calculate some of the placement hints before Technology Mapping and determine if a given cell can be placed by the TechMapper. The JHDL placement grid also allows the use of ints instead of strings in the place() calls, which simplifies the programming process. For example, using the JHDL grid the place() calls from above for XC4000 would be:

    place(Cell Sum, 0, 0, ".F");
    place(Cell Carry, 0, 0, ".G");

The conversion from a JHDL placement grid to XC4000 and Virtex is illustrated below:

Placement Standards

In order to eliminate confusion, reduce complexity, and catch more errors, several placement standards exist in JHDL. They are:

The location of the placement origin is fixed at the upper-left corner of a cell's bounding box. So if the cell is placed at (0,0), the upper-left corner of the cell is what is placed at (0,0). The boundaries of the bounding box are computed just before placing a cell so as to include all of the cell's logic blocks, as shown in the figure below. Thus, when a cell is placed, the whole bounding box is placed. When any other cells are placed relative to an already placed cell, they are placed relative to each other's bounding box.

The placement of a cell's children may not be changed once it is placed, unless the layout is transformed in a predefined manner. This is referred to as a locked placement and it prevents circumvention of placement checking and computation of bounding box. Placement transformations will be described later on.
Multi-level library cells have a standard layout in each technology.

In Xilinx libraries this standard is to have cells laid out in:

Vertical columns
LSB on bottom, MSB on top
Data flow from left to right

Positional Placement

Positional placement is the term used for placing a cell at a certain numerical location within a layer of hierarchy. In JHDL this is done on a two-dimensional grid of X and Y coordinates shown earlier.

Placement in this fashion can be done in the following way for the Full-Adder example in XC4000.

     or_o(and(a,b), and(a, ci), and(b, ci), sum);
     xor_o(a, b, ci, co);
     map(a, b, ci, sum);
     place(sum, 0, 0, ".F");
     map(a, b, ci, co);
     place(co, 0, 0, ".G");

This code will place the sum logic at X=0, Y=0 with a ".F" string property that will be passed to the TechMapper of choice and interpreted there. The same will be true of the carry logic except it will have the ".G" string. Since the TechMapper is for XC4000, it interprets the strings to put the logic at location (0,0) in the F-LUT and G-LUT, respectively.

Relational Cell Placement

Relational cell placement is the ability to place a cell relative to another based on its width, height, and location. It was developed to simplify placement in many cases. Specifically, it is intended to:

Reduce the complexity of numerical calculations for placement
Increase technology independence among designs
Improve readability and reduce verbosity

Relational cell placement is done by using width, height, and location information about cells to compute a new placement location. This information ( Width, Height, X, Y ) is stored in each cell and can be accessed through the following cell methods:

     getWidth()
     getHeight()
     getX()
     getY()

By themselves, these methods do not accomplish much, but convenience methods such as the Logic place() call use them to make placement much easier. The basic format for relational cell placement is:

    place(Cell curr, Directive dir, Cell prev);

Just as if reading a sentence, this method would place the current cell in the direction dir from a previously placed cell. For example, absolute placement of a registered adder might look something like this:

    Wire sum = add(a,b);
    Wire out = reg(sum);
    place(sum, 0, 0);
    place(out, 1, 0);

However, the same placement could be achieved with relational placement in the following way:

    Wire sum = add(a,b);
    Wire out = reg(sum);
    place(out, RIGHT_OF, sum);

Recall that either a reference to the cell or the output wire of the cell can be used in the place() call to place the cell. Notice that the relational placement does not require knowledge about the width of the register or the adder. These methods can be simply read as "place the register to the right of the adder". The relational methods in Logic will decide where the register should be placed based on the specified direction.

Directives

Direction is specifed with the Directive object. 10 Static Directive objects have been created in Logic to handle the common cases. These ten all follow two basic naming schemes as shown in the table and figure below.

Table 1: Directives in Logic

Naming Scheme 1	Naming Scheme 2	Direction
ABOVE	NORTH_OF	UP
BELOW	SOUTH_OF	DOWN
RIGHT_OF	EAST_OF	TORIGHT
LEFT_OF	WEST_OF	TOLEFT
ON	ONTOP_OF	ONTOP

Placement Directives on JHDL Grid

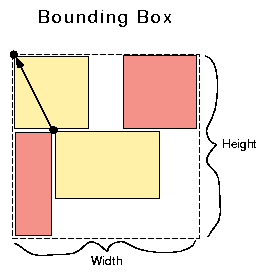
When a relational placement method like the one below is used, several steps are followed to place Cell1.

     place(Cell1, Directive, Cell2);

Compute the bounding box of Cell2 if unknown.
Place Cell2 at X=0, Y=0 if it is unplaced.
Compute bounding box of Cell1.
Compute placement location for Cell1 from widths, heights, X, Y, and Directive.
Place Cell1 and Lock it's placement.

NOTE: Order is important! Remember from the above example that if Cell2 was previously unplaced, it will be placed at X=0,Y=0. If a later call is made to place(Cell3, Directive, Cell4) and Cell4 is not already placed, Cell4 will be placed at X=0, Y=0. This is ON TOP of Cell2, which is probably not what you want. To avoid this, first place Cell4 at a fixed location or relative to Cell1 or Cell2.

Alignment with Directives

All directives have two optional alignment fields. Vertical alignment, which can be used when placing horizontally or in the X direction, and horizontal alignment, which can be used when placing vertically or in the Y direction. When placing a cell on top of another cell, both alignment fields will be used since neither the X nor the Y direction is being specified. Each of the two alignment fields can have any one of three values.

To show how this is done, consider the alignment methods provided by the Directive class:

Vertical Alignment

.alignTop()
.alignCenter()
.alignBottom()

Horizontal Alignment

.alignLeft()
.alignCenter()
.alignRight()

Here is an example of how these alignment directives would be used:

     place( CellA, RIGHT_OF.alignTop(), CellB);

The above method would thus read "place CellA to the right of CellB such that top edges are aligned". If Directives are used without these alignment methods, then the default will be to align left edges, top edges, or both. The following figure illustrates the positioning of the alignment directives:

Placement Directives and Alignments
$\begin{figure}\begin{center}\epsfig{file=../figures/NewPlacement1.eps,height=7.5in,angle=-0} \end{center} \end{figure}$

Multiply Accumulate Example

As an example, consider the design of a multiply accumulate that has a variable width and length. If we wanted to place the accumulator to the right of the multiplier, we could do the following, regardless of what the dimensions of the cells are:

     Cell multiplier = new arrayMult(this, OperandA, OperandB, Clk_en, Product, signed, fully_pipelined);
     place(multiplier, 0, 0);

     Cell accumulator = new accum(this, Product, Clear, Add, Clk_en, Total);
     place(accumulator, RIGHT_OF, multiplier);

The result would look something like this in the XC4000 Layout viewer:

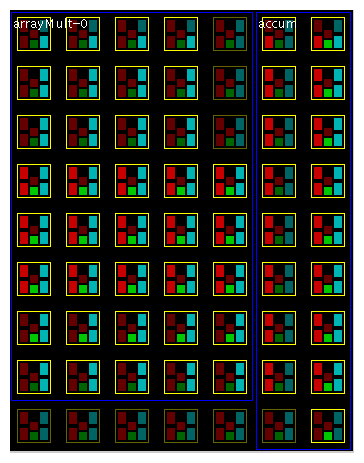
The blue lines in the above figure indicate the bounding boxes and the text indicates the names of the placed cells. As expected, the accumulator was placed to the right of the array multiplier.

Cell Transformations

Cell transformations refer to the group of methods in Logic that change a Cell's layout or placement while preserving its functionality. Cell transformations provide users with different layouts while preserving connectivity of a cell or module. They allow users to change data-flow direction, wrap linear data paths, match port pitch, and move relative to other cells.

Currently, 3 transformations exist: scale, rotate, and translate . They are all methods in the logic class and will accept either cells or output wires as their parameters.

Scale

scale(cell C, int xFact, int yFact);

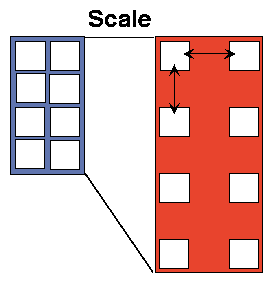
The scale method is designed to stretch a cell's layout by xFact in the x direction and yFact in the y direction. It works by recursively moving children cells to the new position x = x*xFact, y = y*yFact and then recomputing the cell's bounding box. This scaling of the layout can only be done before a cell is placed.

Example

Wire d = wire(11);
Wire q = wire(11);
Cell regbank = new regBank(this, d, q);
scale(regbank, 1, -1);
place(regbank, 0, 0);

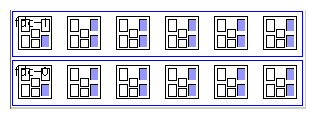
The figures below show the following (in-use registers are marked by blue squares):

The original register bank
The register bank scaled by -1 in the Y direction (notice the CLBs with the single register are now on the bottom)
The register bank scaled by 2 in the X direction
The register bank scaled by 2 in the Y direction

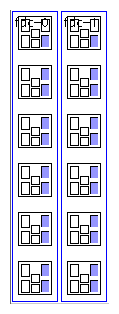
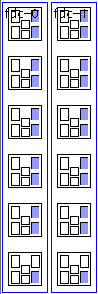
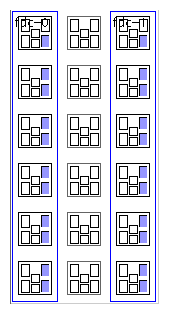
LIMITATIONS

Currently, the scale method only accepts integer factors. Great care should also be taken to ensure that asymmetric features of the FPGA are not scaled, as this will cause an error in the back-end compilation.

Rotate

rotate(cell C, int deg);

The rotate method rotates a Cell's layout by deg degrees in a counter-clockwise direction. It is implemented by recursively moving children to a new position, and then recomputing each cell's bounding box. Again, the rotate method is only valid on a cell that has not yet been placed and has many of the same limitations as scale.

ROTATION by 0, 90, 180, 270 degrees

Example

As an example let's say we wanted to rotate the previous register bank by 90 degrees in the counter-clockwise direction. We could use:

Wire d = wire(11);
Wire q = wire(11);
Cell regbank = new regBank(this, d, q);
rotate(regbank, 90);
place(regbank, 0, 0);

The result would look like this in XC4000 (in-use registers are marked by blue squares):

Translate

translate(cell C, int dx, int dy);

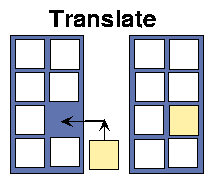
The translate method allows users to move a Cell by dx and dy from it current position. It has the exact same effect as using place(x+dx, y+dy); where x and y are the cell's current placement position. Unlike scale and rotate, translate can only be used after a cell is placed.

Example

Assume that there is a known hole in a module as shown in the figure above. The translate method could be used to fill that hole with a register as shown through the code below.

new Mod1(this, in1, in2, out);
Wire delay = reg(out);
place(Mod1, 0, 0);
place(delay, RIGHT_OF.alignBottom(), out);
translate(delay, -1, -1);

Relational Port Placement

As we have seen thus far, relational cell placement can be very useful for minimizing the FPGA area consumed by a design. Relational Port Placement is designed to minimize delay through the circuitry by aligning ports, or wires.

Verbose Port Placement Method

place(Cell currcell, Wire inWire, Directive dir, Cell prevcell, Wire outWire);

This is the most verbose method for relational port placement. It directs Logic to place currcell in the direction of dir from prevcell, aligning inWire and outWire.
The steps to doing this relational port placement are as follows.

Find port location in prevcell.

Find the leaf cell associated with the alignment wire (source) and ignore cells that cannot be placed.
Compute it's absolute location within the parent.

Find port location in currcell.
Place relationally aligning port locations.

Convenience Methods

place(cell1, dir.align(Wire), cell2);

To eliminate most of the verbosity of the relational port placement method, relational port placement can be done through the relational cell placement methods. This is done by using alignment methods on the Directive which specify the wire to align the two cells with.

To illustrate consider the placement of a registered adder.

Wire sum = add(a,b);
Wire out = reg(sum);
place(out, ONTOP_OF.align(sum), sum);

The above code segment would place the adder at X=0, Y=0 and then place the register in the same location as the adder so that the sum wire fed directly out of the adder and into the register.

Port Placement and Alignment

Since Multi-bit ports with different pitch cannot be perfectly aligned without using the scale method, Alignment methods on Directives were created to specify which bit of the port wire to align. These methods are:

.alignCenter(Wire w) [default]
.alignLSB(Wire w)
.alignMSB(Wire w)

Summary

JHDL Placement reduces complexity.
Low level designs are easier to place.
Parameterized placement of modules is much easier.
Placement of large designs is now feasible.
JHDL Placement improves readability and eliminates verbosity.
Most JHDL Placement can be shared across technologies.
Convenience methods and error checking reduces design time.
Designers can spend more time finding an optimum layout and mapping, without dwelling on the semantics of the language.
Iteration on a designs technology mapping and placment is now possible.

JHDL 0.3.45